Cuboctahedron
| Cuboctahedron | |
|---|---|
 | |
| Type | Archimedean solid |
| Faces | 14 |
| Edges | 24 |
| Vertices | 12 |
| Schläfli symbol | |
| Symmetry group | Octahedral symmetry Tetrahedral symmetry |
| Dihedral angle (degrees) | approximately 125° |
| Dual polyhedron | Rhombic dodecahedron |
| Properties | convex, vector equilibrium, Rupert property |
| Vertex figure | |
 | |
| Net | |
 | |
A cuboctahedron is a polyhedron with 8 triangular faces and 6 square faces. A cuboctahedron has 12 identical vertices, with 2 triangles and 2 squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such, it is a quasiregular polyhedron, i.e., an Archimedean solid that is not only vertex-transitive but also edge-transitive.[1] It is radially equilateral. Its dual polyhedron is the rhombic dodecahedron.
Construction
[edit]The cuboctahedron can be constructed in many ways:
- Its construction can be started by attaching two regular triangular cupolas base-to-base. This is similar to one of the Johnson solids, triangular orthobicupola. The difference is that the triangular orthobicupola is constructed with one of the cupolas twisted so that similar polygonal faces are adjacent, whereas the cuboctahedron is not. As a result, the cuboctahedron may also called the triangular gyrobicupola.[2]
- Its construction can be started from a cube or a regular octahedron, marking the midpoints of their edges, and cutting off all the vertices at those points. This process is known as rectification, making the cuboctahedron being named the rectified cube and rectified octahedron.[3]
- An alternative construction is by cutting of all of the vertices, known as truncation. can be started from a regular tetrahedron, cutting off the vertices and beveling the edges. This process known as cantellation, making the cuboctahedron being named cantellated tetrahedron.[4]
From all of these constructions, the cuboctahedron has 14 faces: 8 equilateral triangles and 6 squares. It also has 24 edges and 12 vertices.[5]
The Cartesian coordinates for the vertices of a cuboctahedron with edge length centered at the origin are:[6]
Properties
[edit]Measurement and other metric properties
[edit]The surface area of a cuboctahedron can be determined by summing all the area of its polygonal faces. The volume of a cuboctahedron can be determined by slicing it off into two regular triangular cupolas, summing up their volume. Given that the edge length , its surface area and volume are:[5]
The dihedral angle of a cuboctahedron can be calculated with the angle of triangular cupolas. The dihedral angle of a triangular cupola between square-to-triangle is approximately 125°, that between square-to-hexagon is 54.7°, and that between triangle-to-hexagon is 70.5°. Therefore, the dihedral angle of a cuboctahedron between square-to-triangle, on the edge where the base of two triangular cupolas are attached is 54.7° + 70.5° approximately 125°. Therefore, the dihedral angle of a cuboctahedron between square-to-triangle is approximately 125°.[7]

Buckminster Fuller found that the cuboctahedron is the only polyhedron in which the distance between its center to the vertex is the same as the distance between its edges. In other words, it has the same length vectors in three-dimensional space, known as vector equilibrium.[8] The rigid struts and the flexible vertices of a cuboctahedron may also be transformed progressively into a regular icosahedron, regular octahedron, regular tetrahedron. Fuller named this the jitterbug transformation.[9]
A cuboctahedron has the Rupert property, meaning there is a polyhedron of the same or larger size that can pass through its hole.[10]
Symmetry and classification
[edit]
The cuboctahedron is an Archimedean solid, meaning it is a highly symmetric and semi-regular polyhedron, and two or more different regular polygonal faces meet in a vertex.[11] The cuboctahedron has two symmetries, resulting from the constructions as has mentioned above: the same symmetry as the regular octahedron or cube, the octahedral symmetry , and the same symmetry as the regular tetrahedron, tetrahedral symmetry .[12] The polygonal faces that meet for every vertex are two equilateral triangles and two squares, and the vertex figure of a cuboctahedron is . The dual of a cuboctahedron is rhombic dodecahedron.[13]
Radial equilateral symmetry
[edit]In a cuboctahedron, the long radius (center to vertex) is the same as the edge length; thus its long diameter (vertex to opposite vertex) is 2 edge lengths. Its center is like the apical vertex of a canonical pyramid: one edge length away from all the other vertices. (In the case of the cuboctahedron, the center is in fact the apex of 6 square and 8 triangular pyramids). This radial equilateral symmetry is a property of only a few uniform polytopes, including the two-dimensional hexagon, the three-dimensional cuboctahedron, and the four-dimensional 24-cell and 8-cell (tesseract). Radially equilateral polytopes are those that can be constructed, with their long radii, from equilateral triangles which meet at the center of the polytope, each contributing two radii and an edge. Therefore, all the interior elements which meet at the center of these polytopes have equilateral triangle inward faces, as in the dissection of the cuboctahedron into 6 square pyramids and 8 tetrahedra.
Each of these radially equilateral polytopes also occurs as cells of a characteristic space-filling tessellation: the tiling of regular hexagons, the rectified cubic honeycomb (of alternating cuboctahedra and octahedra), the 24-cell honeycomb and the tesseractic honeycomb, respectively. Each tessellation has a dual tessellation; the cell centers in a tessellation are cell vertices in its dual tessellation. The densest known regular sphere-packing in two, three and four dimensions uses the cell centers of one of these tessellations as sphere centers.
Because it is radially equilateral, the cuboctahedron's center is one edge length distant from the 12 vertices.
Related polyhedra and honeycomb
[edit]The cuboctahedron shares its skeleton with the two nonconvex uniform polyhedra, the cubohemioctahedron and octahemioctahedron. These polyhedrons are constructed from the skeleton of a cuboctahedron in which the four hexagonal planes bisect its diagonal, intersecting its interior. Adding six squares or eight equilateral triangles results in the cubohemicotahedron or octahemioctahedron, respectively.[14]
The cuboctahedron 2-covers the tetrahemihexahedron, which accordingly has the same abstract vertex figure (two triangles and two squares: ) and half the vertices, edges, and faces. (The actual vertex figure of the tetrahemihexahedron is , with the factor due to the cross.)[15]
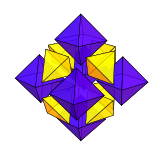
The cuboctahedron can be dissected into 6 square pyramids and 8 tetrahedra meeting at a central point. This dissection is expressed in the tetrahedral-octahedral honeycomb where pairs of square pyramids are combined into octahedra.[16]
Graph
[edit]
The skeleton of a cuboctahedron may be represented as the graph, one of the Archimedean graph. It has 12 vertices and 24 edges. It is quartic graph, which is four vertices connecting each vertex.[17]
The graph of a cuboctahedron may be constructed as the line graph of the cube, making it becomes the locally linear graph.[18]
Appearance
[edit]The cuboctahedron was probably known to Plato: Heron's Definitiones quotes Archimedes as saying that Plato knew of a solid made of 8 triangles and 6 squares.[19]
References
[edit]Footnotes
[edit]- ^ Coxeter 1973, pp. 18–19, §2.3 Quasi-regular polyhedra.
- ^
- ^ van Leeuwen, Freixa & Cano 2023, p. 50.
- ^ Linti 2013, p. 41.
- ^ a b Berman 1971.
- ^ Coxeter 1973, p. 52, §3.7 Coordinates for the vertices of the regular and quasi-regular solids.
- ^ Johnson 1966.
- ^ Cockram 2020, p. 53.
- ^ Verheyen 1989.
- ^ Chai, Yuan & Zamfirescu 2018.
- ^ Diudea 2018, p. 39.
- ^
- Koca & Koca (2013), p. 48
- Cromwell (1997). For octahedral symmetry, see p. 378, Figure 10.13. For tetrahedral symmetry, see p. 380, Figure 10.15.
- ^ Williams 1979, p. 74.
- ^
- ^ Grünbaum 2003, p. 338.
- ^ Posamentier et al. 2022, p. 233–235.
- ^ Read & Wilson 1998, p. 269.
- ^ Fan 1996.
- ^ Turnball 1931.
Works cited
[edit]- Barnes, J. (2012). Gems of Geometry. Springer. doi:10.1007/978-3-642-30964-9. ISBN 978-3-642-30964-9.
- Berman, Martin (1971). "Regular-faced convex polyhedra". Journal of the Franklin Institute. 291 (5): 329–352. doi:10.1016/0016-0032(71)90071-8. MR 0290245.
- Chai, Ying; Yuan, Liping; Zamfirescu, Tudor (2018). "Rupert Property of Archimedean Solids". The American Mathematical Monthly. 125 (6): 497–504. doi:10.1080/00029890.2018.1449505. S2CID 125508192.
- Cockram, Bernice (2020). In Focus Sacred Geometry: Your Personal Guide. Wellfleet Press. ISBN 978-1-57715-225-5.
- Coxeter, H.S.M. (1973) [1948]. Regular Polytopes (3rd ed.). New York: Dover Publications.
- Cromwell, Peter R. (1997), Polyhedra, Cambridge University Press, ISBN 978-0-521-55432-9
- Diudea, M. V. (2018). Multi-shell Polyhedral Clusters. Carbon Materials: Chemistry and Physics. Vol. 10. Springer. doi:10.1007/978-3-319-64123-2. ISBN 978-3-319-64123-2.
- Fan, Cong (1996). "On generalized cages". Journal of Graph Theory. 23 (1): 21–31. doi:10.1002/(SICI)1097-0118(199609)23:1<21::AID-JGT2>3.0.CO;2-M. MR 1402135.
- Ghyka, Matila (1977). The geometry of art and life ([Nachdr.] ed.). New York: Dover Publications. pp. 51–56, 81–84. ISBN 9780486235424.
- Grünbaum, Branko (2003). ""New" uniform polyhedra". In Bezdek, Andras (ed.). Discrete Geometry. CRC Press. ISBN 9780203911211.
- Johnson, Norman W. (1966). "Convex polyhedra with regular faces". Canadian Journal of Mathematics. 18: 169–200. doi:10.4153/cjm-1966-021-8. MR 0185507. S2CID 122006114. Zbl 0132.14603.
- Koca, M.; Koca, N. O. (2013). "Coxeter groups, quaternions, symmetries of polyhedra and 4D polytopes". Mathematical Physics: Proceedings of the 13th Regional Conference, Antalya, Turkey, 27–31 October 2010. World Scientific.
- Linti, G. (2013). "Catenated Compounds - Group 13 [Al, Ga, In, Tl]". In Reedijk, J.; Poeppelmmeier, K. (eds.). Comprehensive Inorganic Chemistry II: From Elements to Applications. Newnes.
- Ogievetsky, O.; Shlosman, S. (2021). "Platonic compounds and cylinders". In Novikov, S.; Krichever, I.; Ogievetsky, O.; Shlosman, S. (eds.). Integrability, Quantization, and Geometry: II. Quantum Theories and Algebraic Geometry. American Mathematical Society. ISBN 978-1-4704-5592-7.
- Pisanski, T.; Servatius, B. (2013). Configuration from a Graphical Viewpoint. Springer. doi:10.1007/978-0-8176-8364-1. ISBN 978-0-8176-8363-4.
- Posamentier, A. S.; Thaller, B.; Dorner, C.; Geretschläger, R.; Maresch, G.; Spreitzer, C.; Stuhlpfarrer, D. (2022). Geometry In Our Three-dimensional World. World Scientific.
- Read, R. C.; Wilson, R. J. (1998). An Atlas of Graphs. Oxford University Press.
- Turnball, H. W. (1931). "A manual of Greek mathematics". Nature. 128 (3235): 739–740. Bibcode:1931Natur.128..739T. doi:10.1038/128739a0. S2CID 3994109.
- van Leeuwen, P.; Freixa, Z.; Cano, I. (2023). "An introduction to chirality". Enantioselective C-C Bond Forming Reactions: From Metal Complex-, Organo-, and Bio-catalyzed Perspectives. Academic Press. ISBN 978-0-443-15774-5.
- Verheyen, H. F. (1989). "The complete set of Jitterbug transformers and the analysis of their motion". Computers and Mathematics with Applications. 17 (1–3): 203–250. doi:10.1016/0898-1221(89)90160-0. MR 0994201.
- Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 978-0-486-23729-9.
External links
[edit]- The Uniform Polyhedra
- Virtual Reality Polyhedra The Encyclopedia of Polyhedra
- Weisstein, Eric W., "Cuboctahedron" ("Archimedean solid") at MathWorld.
- The Cuboctahedron on Hexnet a website devoted to hexagon mathematics.
- Klitzing, Richard. "3D convex uniform polyhedra o3x4o - co".
- Editable printable net of a Cuboctahedron with interactive 3D view































