Collection of proofs of equations involving trigonometric functions
Существует несколько эквивалентных способов определения тригонометрических функций , и доказательства тригонометрических тождеств между ними зависят от выбранного определения. Самые старые и элементарные определения основаны на геометрии прямоугольных треугольников . Доказательства, приведенные в этой статье, используют эти определения и, таким образом, применимы к неотрицательным углам, не превышающим прямого угла . Для больших и отрицательных углов см. Тригонометрические функции .
другие доказательства основаны на ряде Тейлора синуса Другие определения и, следовательно , и косинуса или на дифференциальном уравнении f ″ + f = 0 {\displaystyle f''+f=0}
Тригонометрические функции определяют взаимосвязь между длинами сторон и внутренними углами прямоугольного треугольника. Например, синус угла θ определяется как длина противоположной стороны, деленная на длину гипотенузы. Шесть тригонометрических функций определены для каждого действительного числа , за исключением некоторых из них, для углов, которые отличаются от 0 на кратное прямому углу (90°). На диаграмме справа можно увидеть шесть тригонометрических функций θ для углов, меньших прямого угла:
sin θ = o p p o s i t e h y p o t e n u s e = a h {\displaystyle \sin \theta ={\frac {\mathrm {opposite} }{\mathrm {hypotenuse} }}={\frac {a}{h}}} cos θ = a d j a c e n t h y p o t e n u s e = b h {\displaystyle \cos \theta ={\frac {\mathrm {adjacent} }{\mathrm {hypotenuse} }}={\frac {b}{h}}} tan θ = o p p o s i t e a d j a c e n t = a b {\displaystyle \tan \theta ={\frac {\mathrm {opposite} }{\mathrm {adjacent} }}={\frac {a}{b}}} cot θ = a d j a c e n t o p p o s i t e = b a {\displaystyle \cot \theta ={\frac {\mathrm {adjacent} }{\mathrm {opposite} }}={\frac {b}{a}}} sec θ = h y p o t e n u s e a d j a c e n t = h b {\displaystyle \sec \theta ={\frac {\mathrm {hypotenuse} }{\mathrm {adjacent} }}={\frac {h}{b}}} csc θ = h y p o t e n u s e o p p o s i t e = h a {\displaystyle \csc \theta ={\frac {\mathrm {hypotenuse} }{\mathrm {opposite} }}={\frac {h}{a}}} В случае углов, меньших прямого угла, следующие тождества являются прямыми следствиями приведенных выше определений посредством тождества деления.
a b = ( a h ) ( b h ) . {\displaystyle {\frac {a}{b}}={\frac {\left({\frac {a}{h}}\right)}{\left({\frac {b}{h}}\right)}}.} Они остаются действительными для углов больше 90° и для отрицательных углов.
tan θ = o p p o s i t e a d j a c e n t = ( o p p o s i t e h y p o t e n u s e ) ( a d j a c e n t h y p o t e n u s e ) = sin θ cos θ {\displaystyle \tan \theta ={\frac {\mathrm {opposite} }{\mathrm {adjacent} }}={\frac {\left({\frac {\mathrm {opposite} }{\mathrm {hypotenuse} }}\right)}{\left({\frac {\mathrm {adjacent} }{\mathrm {hypotenuse} }}\right)}}={\frac {\sin \theta }{\cos \theta }}} cot θ = a d j a c e n t o p p o s i t e = ( a d j a c e n t a d j a c e n t ) ( o p p o s i t e a d j a c e n t ) = 1 tan θ = cos θ sin θ {\displaystyle \cot \theta ={\frac {\mathrm {adjacent} }{\mathrm {opposite} }}={\frac {\left({\frac {\mathrm {adjacent} }{\mathrm {adjacent} }}\right)}{\left({\frac {\mathrm {opposite} }{\mathrm {adjacent} }}\right)}}={\frac {1}{\tan \theta }}={\frac {\cos \theta }{\sin \theta }}} sec θ = 1 cos θ = h y p o t e n u s e a d j a c e n t {\displaystyle \sec \theta ={\frac {1}{\cos \theta }}={\frac {\mathrm {hypotenuse} }{\mathrm {adjacent} }}} csc θ = 1 sin θ = h y p o t e n u s e o p p o s i t e {\displaystyle \csc \theta ={\frac {1}{\sin \theta }}={\frac {\mathrm {hypotenuse} }{\mathrm {opposite} }}} tan θ = o p p o s i t e a d j a c e n t = ( o p p o s i t e × h y p o t e n u s e o p p o s i t e × a d j a c e n t ) ( a d j a c e n t × h y p o t e n u s e o p p o s i t e × a d j a c e n t ) = ( h y p o t e n u s e a d j a c e n t ) ( h y p o t e n u s e o p p o s i t e ) = sec θ csc θ {\displaystyle \tan \theta ={\frac {\mathrm {opposite} }{\mathrm {adjacent} }}={\frac {\left({\frac {\mathrm {opposite} \times \mathrm {hypotenuse} }{\mathrm {opposite} \times \mathrm {adjacent} }}\right)}{\left({\frac {\mathrm {adjacent} \times \mathrm {hypotenuse} }{\mathrm {opposite} \times \mathrm {adjacent} }}\right)}}={\frac {\left({\frac {\mathrm {hypotenuse} }{\mathrm {adjacent} }}\right)}{\left({\frac {\mathrm {hypotenuse} }{\mathrm {opposite} }}\right)}}={\frac {\sec \theta }{\csc \theta }}} Или
tan θ = sin θ cos θ = ( 1 csc θ ) ( 1 sec θ ) = ( csc θ sec θ csc θ ) ( csc θ sec θ sec θ ) = sec θ csc θ {\displaystyle \tan \theta ={\frac {\sin \theta }{\cos \theta }}={\frac {\left({\frac {1}{\csc \theta }}\right)}{\left({\frac {1}{\sec \theta }}\right)}}={\frac {\left({\frac {\csc \theta \sec \theta }{\csc \theta }}\right)}{\left({\frac {\csc \theta \sec \theta }{\sec \theta }}\right)}}={\frac {\sec \theta }{\csc \theta }}} cot θ = csc θ sec θ {\displaystyle \cot \theta ={\frac {\csc \theta }{\sec \theta }}} Два угла, сумма которых равна π/2 радиан (90 градусов), являются дополнительными . На диаграмме углы в вершинах A и B дополняют друг друга, поэтому мы можем поменять местами a и b и изменить θ на π/2 − θ, получив:
sin ( π / 2 − θ ) = cos θ {\displaystyle \sin \left(\pi /2-\theta \right)=\cos \theta } cos ( π / 2 − θ ) = sin θ {\displaystyle \cos \left(\pi /2-\theta \right)=\sin \theta } tan ( π / 2 − θ ) = cot θ {\displaystyle \tan \left(\pi /2-\theta \right)=\cot \theta } cot ( π / 2 − θ ) = tan θ {\displaystyle \cot \left(\pi /2-\theta \right)=\tan \theta } sec ( π / 2 − θ ) = csc θ {\displaystyle \sec \left(\pi /2-\theta \right)=\csc \theta } csc ( π / 2 − θ ) = sec θ {\displaystyle \csc \left(\pi /2-\theta \right)=\sec \theta } Идентичность 1:
sin 2 θ + cos 2 θ = 1 {\displaystyle \sin ^{2}\theta +\cos ^{2}\theta =1} Отсюда и тождества отношений следуют два следующих результата. Чтобы получить первое, разделите обе части sin 2 θ + cos 2 θ = 1 {\displaystyle \sin ^{2}\theta +\cos ^{2}\theta =1} cos 2 θ {\displaystyle \cos ^{2}\theta } sin 2 θ {\displaystyle \sin ^{2}\theta }
tan 2 θ + 1 = sec 2 θ {\displaystyle \tan ^{2}\theta +1\ =\sec ^{2}\theta } sec 2 θ − tan 2 θ = 1 {\displaystyle \sec ^{2}\theta -\tan ^{2}\theta =1} Сходным образом
1 + cot 2 θ = csc 2 θ {\displaystyle 1\ +\cot ^{2}\theta =\csc ^{2}\theta } csc 2 θ − cot 2 θ = 1 {\displaystyle \csc ^{2}\theta -\cot ^{2}\theta =1} Идентичность 2:
Следующее объясняет все три взаимные функции.
csc 2 θ + sec 2 θ − cot 2 θ = 2 + tan 2 θ {\displaystyle \csc ^{2}\theta +\sec ^{2}\theta -\cot ^{2}\theta =2\ +\tan ^{2}\theta } Доказательство 2:
Обратитесь к диаграмме треугольника выше. Обратите внимание, что a 2 + b 2 = h 2 {\displaystyle a^{2}+b^{2}=h^{2}} теореме Пифагора .
csc 2 θ + sec 2 θ = h 2 a 2 + h 2 b 2 = a 2 + b 2 a 2 + a 2 + b 2 b 2 = 2 + b 2 a 2 + a 2 b 2 {\displaystyle \csc ^{2}\theta +\sec ^{2}\theta ={\frac {h^{2}}{a^{2}}}+{\frac {h^{2}}{b^{2}}}={\frac {a^{2}+b^{2}}{a^{2}}}+{\frac {a^{2}+b^{2}}{b^{2}}}=2\ +{\frac {b^{2}}{a^{2}}}+{\frac {a^{2}}{b^{2}}}} Замена соответствующими функциями -
2 + b 2 a 2 + a 2 b 2 = 2 + tan 2 θ + cot 2 θ {\displaystyle 2\ +{\frac {b^{2}}{a^{2}}}+{\frac {a^{2}}{b^{2}}}=2\ +\tan ^{2}\theta +\cot ^{2}\theta } Перестановка дает:
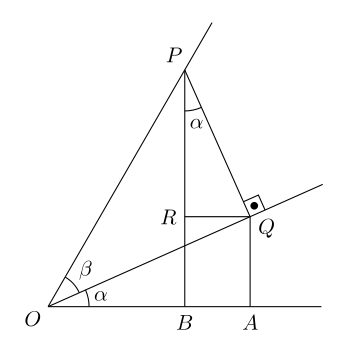
csc 2 θ + sec 2 θ − cot 2 θ = 2 + tan 2 θ {\displaystyle \csc ^{2}\theta +\sec ^{2}\theta -\cot ^{2}\theta =2\ +\tan ^{2}\theta } Иллюстрация формулы суммы. Нарисуйте горизонтальную линию ( ось X ); отметьте начало координат О. Нарисуйте линию от О под углом α {\displaystyle \alpha } β {\displaystyle \beta } осью x равен α + β {\displaystyle \alpha +\beta }
Поместите P на линию, определяемую α + β {\displaystyle \alpha +\beta }
Пусть PQ — линия, перпендикулярная линии OQ, определяемая углом α {\displaystyle \alpha } ∴ {\displaystyle \therefore }
Пусть QA — перпендикуляр из точки A на оси x к Q, а PB — перпендикуляр из точки B на оси x к P. ∴ {\displaystyle \therefore }
Нарисуйте R на PB так, чтобы QR был параллелен оси x .
Теперь угол R P Q = α {\displaystyle RPQ=\alpha } O Q A = π 2 − α {\displaystyle OQA={\frac {\pi }{2}}-\alpha } R Q O = α , R Q P = π 2 − α {\displaystyle RQO=\alpha ,RQP={\frac {\pi }{2}}-\alpha } R P Q = α {\displaystyle RPQ=\alpha }
R P Q = π 2 − R Q P = π 2 − ( π 2 − R Q O ) = R Q O = α {\displaystyle RPQ={\tfrac {\pi }{2}}-RQP={\tfrac {\pi }{2}}-({\tfrac {\pi }{2}}-RQO)=RQO=\alpha } O P = 1 {\displaystyle OP=1} P Q = sin β {\displaystyle PQ=\sin \beta } O Q = cos β {\displaystyle OQ=\cos \beta } A Q O Q = sin α {\displaystyle {\frac {AQ}{OQ}}=\sin \alpha } A Q = sin α cos β {\displaystyle AQ=\sin \alpha \cos \beta } P R P Q = cos α {\displaystyle {\frac {PR}{PQ}}=\cos \alpha } P R = cos α sin β {\displaystyle PR=\cos \alpha \sin \beta } sin ( α + β ) = P B = R B + P R = A Q + P R = sin α cos β + cos α sin β {\displaystyle \sin(\alpha +\beta )=PB=RB+PR=AQ+PR=\sin \alpha \cos \beta +\cos \alpha \sin \beta } Подставив − β {\displaystyle -\beta } β {\displaystyle \beta } тождества отражения четных и нечетных функций , мы также получаем:
sin ( α − β ) = sin α cos ( − β ) + cos α sin ( − β ) {\displaystyle \sin(\alpha -\beta )=\sin \alpha \cos(-\beta )+\cos \alpha \sin(-\beta )} sin ( α − β ) = sin α cos β − cos α sin β {\displaystyle \sin(\alpha -\beta )=\sin \alpha \cos \beta -\cos \alpha \sin \beta } Используя рисунок выше,
O P = 1 {\displaystyle OP=1} P Q = sin β {\displaystyle PQ=\sin \beta } O Q = cos β {\displaystyle OQ=\cos \beta } O A O Q = cos α {\displaystyle {\frac {OA}{OQ}}=\cos \alpha } O A = cos α cos β {\displaystyle OA=\cos \alpha \cos \beta } R Q P Q = sin α {\displaystyle {\frac {RQ}{PQ}}=\sin \alpha } R Q = sin α sin β {\displaystyle RQ=\sin \alpha \sin \beta } cos ( α + β ) = O B = O A − B A = O A − R Q = cos α cos β − sin α sin β {\displaystyle \cos(\alpha +\beta )=OB=OA-BA=OA-RQ=\cos \alpha \cos \beta \ -\sin \alpha \sin \beta } Подставив − β {\displaystyle -\beta } β {\displaystyle \beta } тождества отражения четных и нечетных функций , мы также получаем:
cos ( α − β ) = cos α cos ( − β ) − sin α sin ( − β ) , {\displaystyle \cos(\alpha -\beta )=\cos \alpha \cos(-\beta )-\sin \alpha \sin(-\beta ),} cos ( α − β ) = cos α cos β + sin α sin β {\displaystyle \cos(\alpha -\beta )=\cos \alpha \cos \beta +\sin \alpha \sin \beta } Кроме того, используя дополнительные формулы угла ,
cos ( α + β ) = sin ( π / 2 − ( α + β ) ) = sin ( ( π / 2 − α ) − β ) = sin ( π / 2 − α ) cos β − cos ( π / 2 − α ) sin β = cos α cos β − sin α sin β {\displaystyle {\begin{aligned}\cos(\alpha +\beta )&=\sin \left(\pi /2-(\alpha +\beta )\right)\\&=\sin \left((\pi /2-\alpha )-\beta \right)\\&=\sin \left(\pi /2-\alpha \right)\cos \beta -\cos \left(\pi /2-\alpha \right)\sin \beta \\&=\cos \alpha \cos \beta -\sin \alpha \sin \beta \\\end{aligned}}} Из формул синуса и косинуса получаем
tan ( α + β ) = sin ( α + β ) cos ( α + β ) = sin α cos β + cos α sin β cos α cos β − sin α sin β {\displaystyle \tan(\alpha +\beta )={\frac {\sin(\alpha +\beta )}{\cos(\alpha +\beta )}}={\frac {\sin \alpha \cos \beta +\cos \alpha \sin \beta }{\cos \alpha \cos \beta -\sin \alpha \sin \beta }}} Разделив числитель и знаменатель на cos α cos β {\displaystyle \cos \alpha \cos \beta }
tan ( α + β ) = tan α + tan β 1 − tan α tan β {\displaystyle \tan(\alpha +\beta )={\frac {\tan \alpha +\tan \beta }{1-\tan \alpha \tan \beta }}} Вычитание β {\displaystyle \beta } α {\displaystyle \alpha } tan ( − β ) = − tan β {\displaystyle \tan(-\beta )=-\tan \beta }
tan ( α − β ) = tan α + tan ( − β ) 1 − tan α tan ( − β ) = tan α − tan β 1 + tan α tan β {\displaystyle \tan(\alpha -\beta )={\frac {\tan \alpha +\tan(-\beta )}{1-\tan \alpha \tan(-\beta )}}={\frac {\tan \alpha -\tan \beta }{1+\tan \alpha \tan \beta }}} Аналогично из формул синуса и косинуса получаем
cot ( α + β ) = cos ( α + β ) sin ( α + β ) = cos α cos β − sin α sin β sin α cos β + cos α sin β {\displaystyle \cot(\alpha +\beta )={\frac {\cos(\alpha +\beta )}{\sin(\alpha +\beta )}}={\frac {\cos \alpha \cos \beta -\sin \alpha \sin \beta }{\sin \alpha \cos \beta +\cos \alpha \sin \beta }}} Затем разделив числитель и знаменатель на sin α sin β {\displaystyle \sin \alpha \sin \beta }
cot ( α + β ) = cot α cot β − 1 cot α + cot β {\displaystyle \cot(\alpha +\beta )={\frac {\cot \alpha \cot \beta -1}{\cot \alpha +\cot \beta }}} Или, используя cot θ = 1 tan θ {\displaystyle \cot \theta ={\frac {1}{\tan \theta }}}
cot ( α + β ) = 1 − tan α tan β tan α + tan β = 1 tan α tan β − 1 1 tan α + 1 tan β = cot α cot β − 1 cot α + cot β {\displaystyle \cot(\alpha +\beta )={\frac {1-\tan \alpha \tan \beta }{\tan \alpha +\tan \beta }}={\frac {{\frac {1}{\tan \alpha \tan \beta }}-1}{{\frac {1}{\tan \alpha }}+{\frac {1}{\tan \beta }}}}={\frac {\cot \alpha \cot \beta -1}{\cot \alpha +\cot \beta }}} С использованием cot ( − β ) = − cot β {\displaystyle \cot(-\beta )=-\cot \beta }
cot ( α − β ) = cot α cot ( − β ) − 1 cot α + cot ( − β ) = cot α cot β + 1 cot β − cot α {\displaystyle \cot(\alpha -\beta )={\frac {\cot \alpha \cot(-\beta )-1}{\cot \alpha +\cot(-\beta )}}={\frac {\cot \alpha \cot \beta +1}{\cot \beta -\cot \alpha }}} Из тождеств суммы углов получаем
sin ( 2 θ ) = 2 sin θ cos θ {\displaystyle \sin(2\theta )=2\sin \theta \cos \theta } и
cos ( 2 θ ) = cos 2 θ − sin 2 θ {\displaystyle \cos(2\theta )=\cos ^{2}\theta -\sin ^{2}\theta } Пифагорейские тождества дают две альтернативные формы последнего из них:
cos ( 2 θ ) = 2 cos 2 θ − 1 {\displaystyle \cos(2\theta )=2\cos ^{2}\theta -1} cos ( 2 θ ) = 1 − 2 sin 2 θ {\displaystyle \cos(2\theta )=1-2\sin ^{2}\theta } Тождества суммы углов также дают
tan ( 2 θ ) = 2 tan θ 1 − tan 2 θ = 2 cot θ − tan θ {\displaystyle \tan(2\theta )={\frac {2\tan \theta }{1-\tan ^{2}\theta }}={\frac {2}{\cot \theta -\tan \theta }}} cot ( 2 θ ) = cot 2 θ − 1 2 cot θ = cot θ − tan θ 2 {\displaystyle \cot(2\theta )={\frac {\cot ^{2}\theta -1}{2\cot \theta }}={\frac {\cot \theta -\tan \theta }{2}}} Это также можно доказать, используя формулу Эйлера
e i φ = cos φ + i sin φ {\displaystyle e^{i\varphi }=\cos \varphi +i\sin \varphi } Возведение в квадрат обеих сторон дает
e i 2 φ = ( cos φ + i sin φ ) 2 {\displaystyle e^{i2\varphi }=(\cos \varphi +i\sin \varphi )^{2}} Но замена угла его удвоенной версией, которая дает тот же результат в левой части уравнения, дает
e i 2 φ = cos 2 φ + i sin 2 φ {\displaystyle e^{i2\varphi }=\cos 2\varphi +i\sin 2\varphi } Отсюда следует, что
( cos φ + i sin φ ) 2 = cos 2 φ + i sin 2 φ {\displaystyle (\cos \varphi +i\sin \varphi )^{2}=\cos 2\varphi +i\sin 2\varphi } Расширение квадрата и упрощение левой части уравнения дает
i ( 2 sin φ cos φ ) + cos 2 φ − sin 2 φ = cos 2 φ + i sin 2 φ {\displaystyle i(2\sin \varphi \cos \varphi )+\cos ^{2}\varphi -\sin ^{2}\varphi \ =\cos 2\varphi +i\sin 2\varphi } Поскольку мнимая и реальная части должны быть одинаковыми, у нас остаются исходные тождества.
cos 2 φ − sin 2 φ = cos 2 φ {\displaystyle \cos ^{2}\varphi -\sin ^{2}\varphi \ =\cos 2\varphi } а также
2 sin φ cos φ = sin 2 φ {\displaystyle 2\sin \varphi \cos \varphi =\sin 2\varphi } Два тождества, дающие альтернативные формы для cos 2θ, приводят к следующим уравнениям:
cos θ 2 = ± 1 + cos θ 2 , {\displaystyle \cos {\frac {\theta }{2}}=\pm \,{\sqrt {\frac {1+\cos \theta }{2}}},} sin θ 2 = ± 1 − cos θ 2 . {\displaystyle \sin {\frac {\theta }{2}}=\pm \,{\sqrt {\frac {1-\cos \theta }{2}}}.} Знак квадратного корня необходимо выбрать правильно - обратите внимание, что если к θ добавить 2 π , величины внутри квадратных корней не изменятся, но левые части уравнений меняют знак. Следовательно, правильный знак зависит от значения θ.
Для функции tan уравнение имеет вид:
tan θ 2 = ± 1 − cos θ 1 + cos θ . {\displaystyle \tan {\frac {\theta }{2}}=\pm \,{\sqrt {\frac {1-\cos \theta }{1+\cos \theta }}}.} Тогда умножение числителя и знаменателя внутри квадратного корня на (1 + cos θ) и использование тождеств Пифагора приводит к:
tan θ 2 = sin θ 1 + cos θ . {\displaystyle \tan {\frac {\theta }{2}}={\frac {\sin \theta }{1+\cos \theta }}.} Кроме того, если числитель и знаменатель умножаются на (1 - cos θ), результат будет:
tan θ 2 = 1 − cos θ sin θ . {\displaystyle \tan {\frac {\theta }{2}}={\frac {1-\cos \theta }{\sin \theta }}.} Это также дает:
tan θ 2 = csc θ − cot θ . {\displaystyle \tan {\frac {\theta }{2}}=\csc \theta -\cot \theta .} Аналогичные манипуляции для функции раскладушки дают:
cot θ 2 = ± 1 + cos θ 1 − cos θ = 1 + cos θ sin θ = sin θ 1 − cos θ = csc θ + cot θ . {\displaystyle \cot {\frac {\theta }{2}}=\pm \,{\sqrt {\frac {1+\cos \theta }{1-\cos \theta }}}={\frac {1+\cos \theta }{\sin \theta }}={\frac {\sin \theta }{1-\cos \theta }}=\csc \theta +\cot \theta .} Если ψ + θ + ϕ = π = {\displaystyle \psi +\theta +\phi =\pi =} ψ {\displaystyle \psi } θ {\displaystyle \theta } ϕ {\displaystyle \phi }
tan ( ψ ) + tan ( θ ) + tan ( ϕ ) = tan ( ψ ) tan ( θ ) tan ( ϕ ) . {\displaystyle \tan(\psi )+\tan(\theta )+\tan(\phi )=\tan(\psi )\tan(\theta )\tan(\phi ).} Доказательство: [1]
ψ = π − θ − ϕ tan ( ψ ) = tan ( π − θ − ϕ ) = − tan ( θ + ϕ ) = − tan θ − tan ϕ 1 − tan θ tan ϕ = tan θ + tan ϕ tan θ tan ϕ − 1 ( tan θ tan ϕ − 1 ) tan ψ = tan θ + tan ϕ tan ψ tan θ tan ϕ − tan ψ = tan θ + tan ϕ tan ψ tan θ tan ϕ = tan ψ + tan θ + tan ϕ {\displaystyle {\begin{aligned}\psi &=\pi -\theta -\phi \\\tan(\psi )&=\tan(\pi -\theta -\phi )\\&=-\tan(\theta +\phi )\\&={\frac {-\tan \theta -\tan \phi }{1-\tan \theta \tan \phi }}\\&={\frac {\tan \theta +\tan \phi }{\tan \theta \tan \phi -1}}\\(\tan \theta \tan \phi -1)\tan \psi &=\tan \theta +\tan \phi \\\tan \psi \tan \theta \tan \phi -\tan \psi &=\tan \theta +\tan \phi \\\tan \psi \tan \theta \tan \phi &=\tan \psi +\tan \theta +\tan \phi \\\end{aligned}}} Если ψ + θ + ϕ = π 2 = {\displaystyle \psi +\theta +\phi ={\tfrac {\pi }{2}}=}
cot ( ψ ) + cot ( θ ) + cot ( ϕ ) = cot ( ψ ) cot ( θ ) cot ( ϕ ) {\displaystyle \cot(\psi )+\cot(\theta )+\cot(\phi )=\cot(\psi )\cot(\theta )\cot(\phi )} Доказательство:
Замените каждый из ψ {\displaystyle \psi } θ {\displaystyle \theta } ϕ {\displaystyle \phi }
Данный
ψ + θ + ϕ = π 2 {\displaystyle \psi +\theta +\phi ={\tfrac {\pi }{2}}} ∴ ( π 2 − ψ ) + ( π 2 − θ ) + ( π 2 − ϕ ) = 3 π 2 − ( ψ + θ + ϕ ) = 3 π 2 − π 2 = π {\displaystyle \therefore ({\tfrac {\pi }{2}}-\psi )+({\tfrac {\pi }{2}}-\theta )+({\tfrac {\pi }{2}}-\phi )={\tfrac {3\pi }{2}}-(\psi +\theta +\phi )={\tfrac {3\pi }{2}}-{\tfrac {\pi }{2}}=\pi } так что результат следует из тождества тройного касания.
sin θ ± sin ϕ = 2 sin ( θ ± ϕ 2 ) cos ( θ ∓ ϕ 2 ) {\displaystyle \sin \theta \pm \sin \phi =2\sin \left({\frac {\theta \pm \phi }{2}}\right)\cos \left({\frac {\theta \mp \phi }{2}}\right)} cos θ + cos ϕ = 2 cos ( θ + ϕ 2 ) cos ( θ − ϕ 2 ) {\displaystyle \cos \theta +\cos \phi =2\cos \left({\frac {\theta +\phi }{2}}\right)\cos \left({\frac {\theta -\phi }{2}}\right)} cos θ − cos ϕ = − 2 sin ( θ + ϕ 2 ) sin ( θ − ϕ 2 ) {\displaystyle \cos \theta -\cos \phi =-2\sin \left({\frac {\theta +\phi }{2}}\right)\sin \left({\frac {\theta -\phi }{2}}\right)} Во-первых, начнем с тождеств суммы и угла:
sin ( α + β ) = sin α cos β + cos α sin β {\displaystyle \sin(\alpha +\beta )=\sin \alpha \cos \beta +\cos \alpha \sin \beta } sin ( α − β ) = sin α cos β − cos α sin β {\displaystyle \sin(\alpha -\beta )=\sin \alpha \cos \beta -\cos \alpha \sin \beta } Сложив их вместе,
sin ( α + β ) + sin ( α − β ) = sin α cos β + cos α sin β + sin α cos β − cos α sin β = 2 sin α cos β {\displaystyle \sin(\alpha +\beta )+\sin(\alpha -\beta )=\sin \alpha \cos \beta +\cos \alpha \sin \beta +\sin \alpha \cos \beta -\cos \alpha \sin \beta =2\sin \alpha \cos \beta } Аналогично, вычитая два тождества суммы и угла,
sin ( α + β ) − sin ( α − β ) = sin α cos β + cos α sin β − sin α cos β + cos α sin β = 2 cos α sin β {\displaystyle \sin(\alpha +\beta )-\sin(\alpha -\beta )=\sin \alpha \cos \beta +\cos \alpha \sin \beta -\sin \alpha \cos \beta +\cos \alpha \sin \beta =2\cos \alpha \sin \beta } Позволять α + β = θ {\displaystyle \alpha +\beta =\theta } α − β = ϕ {\displaystyle \alpha -\beta =\phi }
∴ α = θ + ϕ 2 {\displaystyle \therefore \alpha ={\frac {\theta +\phi }{2}}} β = θ − ϕ 2 {\displaystyle \beta ={\frac {\theta -\phi }{2}}} Заменять θ {\displaystyle \theta } ϕ {\displaystyle \phi }
sin θ + sin ϕ = 2 sin ( θ + ϕ 2 ) cos ( θ − ϕ 2 ) {\displaystyle \sin \theta +\sin \phi =2\sin \left({\frac {\theta +\phi }{2}}\right)\cos \left({\frac {\theta -\phi }{2}}\right)} sin θ − sin ϕ = 2 cos ( θ + ϕ 2 ) sin ( θ − ϕ 2 ) = 2 sin ( θ − ϕ 2 ) cos ( θ + ϕ 2 ) {\displaystyle \sin \theta -\sin \phi =2\cos \left({\frac {\theta +\phi }{2}}\right)\sin \left({\frac {\theta -\phi }{2}}\right)=2\sin \left({\frac {\theta -\phi }{2}}\right)\cos \left({\frac {\theta +\phi }{2}}\right)} Поэтому,
sin θ ± sin ϕ = 2 sin ( θ ± ϕ 2 ) cos ( θ ∓ ϕ 2 ) {\displaystyle \sin \theta \pm \sin \phi =2\sin \left({\frac {\theta \pm \phi }{2}}\right)\cos \left({\frac {\theta \mp \phi }{2}}\right)} Аналогично для косинуса начните с тождеств суммы и угла:
cos ( α + β ) = cos α cos β − sin α sin β {\displaystyle \cos(\alpha +\beta )=\cos \alpha \cos \beta \ -\sin \alpha \sin \beta } cos ( α − β ) = cos α cos β + sin α sin β {\displaystyle \cos(\alpha -\beta )=\cos \alpha \cos \beta +\sin \alpha \sin \beta } Опять же, прибавляя и вычитая
cos ( α + β ) + cos ( α − β ) = cos α cos β − sin α sin β + cos α cos β + sin α sin β = 2 cos α cos β {\displaystyle \cos(\alpha +\beta )+\cos(\alpha -\beta )=\cos \alpha \cos \beta \ -\sin \alpha \sin \beta +\cos \alpha \cos \beta +\sin \alpha \sin \beta =2\cos \alpha \cos \beta } cos ( α + β ) − cos ( α − β ) = cos α cos β − sin α sin β − cos α cos β − sin α sin β = − 2 sin α sin β {\displaystyle \cos(\alpha +\beta )-\cos(\alpha -\beta )=\cos \alpha \cos \beta \ -\sin \alpha \sin \beta -\cos \alpha \cos \beta -\sin \alpha \sin \beta =-2\sin \alpha \sin \beta } Заменять θ {\displaystyle \theta } ϕ {\displaystyle \phi }
cos θ + cos ϕ = 2 cos ( θ + ϕ 2 ) cos ( θ − ϕ 2 ) {\displaystyle \cos \theta +\cos \phi =2\cos \left({\frac {\theta +\phi }{2}}\right)\cos \left({\frac {\theta -\phi }{2}}\right)} cos θ − cos ϕ = − 2 sin ( θ + ϕ 2 ) sin ( θ − ϕ 2 ) {\displaystyle \cos \theta -\cos \phi =-2\sin \left({\frac {\theta +\phi }{2}}\right)\sin \left({\frac {\theta -\phi }{2}}\right)} Иллюстрация синусоидального и касательного неравенств. На рисунке справа показан сектор круга радиусом 1. Этот сектор равен θ /(2 π ) θ /2 θ < π /2
O A = O D = 1 {\displaystyle OA=OD=1} A B = sin θ {\displaystyle AB=\sin \theta } C D = tan θ {\displaystyle CD=\tan \theta } Площадь треугольника OAD AB /2 sin( θ )/2 . Площадь треугольника OCD CD /2 tan( θ )/2 .
Поскольку треугольник OAD OCD
sin θ < θ < tan θ . {\displaystyle \sin \theta <\theta <\tan \theta .} Этот геометрический аргумент основан на определениях длины дуги и area , которые выступают в качестве допущений, поэтому это скорее условие, налагаемое при построении тригонометрических функций, чемдоказуемое свойство. [2] θ > π /2 θ > 1 sin θ ≤ 1 (из-за тождества Пифагора), поэтому sin θ < θ . Итак, у нас есть
sin θ θ < 1 i f 0 < θ . {\displaystyle {\frac {\sin \theta }{\theta }}<1\ \ \ \mathrm {if} \ \ \ 0<\theta .} Для отрицательных значений θ
sin θ θ = sin ( − θ ) − θ < 1. {\displaystyle {\frac {\sin \theta }{\theta }}={\frac {\sin(-\theta )}{-\theta }}<1.} Следовательно
sin θ θ < 1 if θ ≠ 0 , {\displaystyle {\frac {\sin \theta }{\theta }}<1\quad {\text{if }}\quad \theta \neq 0,} и
tan θ θ > 1 if 0 < θ < π 2 . {\displaystyle {\frac {\tan \theta }{\theta }}>1\quad {\text{if }}\quad 0<\theta <{\frac {\pi }{2}}.} lim θ → 0 sin θ = 0 {\displaystyle \lim _{\theta \to 0}{\sin \theta }=0} lim θ → 0 cos θ = 1 {\displaystyle \lim _{\theta \to 0}{\cos \theta }=1} lim θ → 0 sin θ θ = 1 {\displaystyle \lim _{\theta \to 0}{\frac {\sin \theta }{\theta }}=1} Другими словами, функция синус дифференцируема в точке 0, а ее производная равна 1.
Доказательство. Из предыдущих неравенств для малых углов имеем
sin θ < θ < tan θ {\displaystyle \sin \theta <\theta <\tan \theta } Поэтому,
sin θ θ < 1 < tan θ θ {\displaystyle {\frac {\sin \theta }{\theta }}<1<{\frac {\tan \theta }{\theta }}} Рассмотрим правое неравенство. С
tan θ = sin θ cos θ {\displaystyle \tan \theta ={\frac {\sin \theta }{\cos \theta }}} ∴ 1 < sin θ θ cos θ {\displaystyle \therefore 1<{\frac {\sin \theta }{\theta \cos \theta }}} Умножить на cos θ {\displaystyle \cos \theta }
cos θ < sin θ θ {\displaystyle \cos \theta <{\frac {\sin \theta }{\theta }}} В сочетании с левым неравенством:
cos θ < sin θ θ < 1 {\displaystyle \cos \theta <{\frac {\sin \theta }{\theta }}<1} принимая cos θ {\displaystyle \cos \theta } θ → 0 {\displaystyle \theta \to 0}
lim θ → 0 cos θ = 1 {\displaystyle \lim _{\theta \to 0}{\cos \theta }=1} Поэтому,
lim θ → 0 sin θ θ = 1 {\displaystyle \lim _{\theta \to 0}{\frac {\sin \theta }{\theta }}=1} lim θ → 0 1 − cos θ θ = 0 {\displaystyle \lim _{\theta \to 0}{\frac {1-\cos \theta }{\theta }}=0} Доказательство:
1 − cos θ θ = 1 − cos 2 θ θ ( 1 + cos θ ) = sin 2 θ θ ( 1 + cos θ ) = ( sin θ θ ) × sin θ × ( 1 1 + cos θ ) {\displaystyle {\begin{aligned}{\frac {1-\cos \theta }{\theta }}&={\frac {1-\cos ^{2}\theta }{\theta (1+\cos \theta )}}\\&={\frac {\sin ^{2}\theta }{\theta (1+\cos \theta )}}\\&=\left({\frac {\sin \theta }{\theta }}\right)\times \sin \theta \times \left({\frac {1}{1+\cos \theta }}\right)\\\end{aligned}}} Пределы этих трех величин равны 1, 0 и 1/2, поэтому результирующий предел равен нулю.
lim θ → 0 1 − cos θ θ 2 = 1 2 {\displaystyle \lim _{\theta \to 0}{\frac {1-\cos \theta }{\theta ^{2}}}={\frac {1}{2}}} Доказательство:
Как и в предыдущем доказательстве,
1 − cos θ θ 2 = sin θ θ × sin θ θ × 1 1 + cos θ . {\displaystyle {\frac {1-\cos \theta }{\theta ^{2}}}={\frac {\sin \theta }{\theta }}\times {\frac {\sin \theta }{\theta }}\times {\frac {1}{1+\cos \theta }}.} Пределы этих трех величин равны 1, 1 и 1/2, поэтому результирующий предел равен 1/2.
Доказательство композиции тригонометрических и обратных тригонометрических функций. [ редактировать ] Все эти функции следуют из тригонометрического тождества Пифагора. Мы можем доказать, например, функцию
sin [ arctan ( x ) ] = x 1 + x 2 {\displaystyle \sin[\arctan(x)]={\frac {x}{\sqrt {1+x^{2}}}}} Доказательство:
Мы начинаем с
sin 2 θ + cos 2 θ = 1 {\displaystyle \sin ^{2}\theta +\cos ^{2}\theta =1} Затем разделим это уравнение (I) на cos 2 θ {\displaystyle \cos ^{2}\theta }
cos 2 θ = 1 tan 2 θ + 1 {\displaystyle \cos ^{2}\theta ={\frac {1}{\tan ^{2}\theta +1}}} 1 − sin 2 θ = 1 tan 2 θ + 1 {\displaystyle 1-\sin ^{2}\theta ={\frac {1}{\tan ^{2}\theta +1}}} Затем используйте замену θ = arctan ( x ) {\displaystyle \theta =\arctan(x)}
1 − sin 2 [ arctan ( x ) ] = 1 tan 2 [ arctan ( x ) ] + 1 {\displaystyle 1-\sin ^{2}[\arctan(x)]={\frac {1}{\tan ^{2}[\arctan(x)]+1}}} sin 2 [ arctan ( x ) ] = tan 2 [ arctan ( x ) ] tan 2 [ arctan ( x ) ] + 1 {\displaystyle \sin ^{2}[\arctan(x)]={\frac {\tan ^{2}[\arctan(x)]}{\tan ^{2}[\arctan(x)]+1}}} Затем мы используем тождество tan [ arctan ( x ) ] ≡ x {\displaystyle \tan[\arctan(x)]\equiv x}
sin [ arctan ( x ) ] = x x 2 + 1 {\displaystyle \sin[\arctan(x)]={\frac {x}{\sqrt {x^{2}+1}}}} И первоначальная тригонометрическая тождественность Пифагора доказана...
sin 2 θ {\displaystyle \sin ^{2}\theta }
sin 2 θ = 1 1 1 + 1 tan 2 θ {\displaystyle \sin ^{2}\theta ={\frac {\frac {1}{1}}{1+{\frac {1}{\tan ^{2}\theta }}}}} sin 2 θ = tan 2 θ tan 2 θ + 1 {\displaystyle \sin ^{2}\theta ={\frac {\tan ^{2}\theta }{\tan ^{2}\theta +1}}} Затем используйте замену θ = arctan ( x ) {\displaystyle \theta =\arctan(x)}
sin 2 [ arctan ( x ) ] = tan 2 [ arctan ( x ) ] tan 2 [ arctan ( x ) ] + 1 {\displaystyle \sin ^{2}[\arctan(x)]={\frac {\tan ^{2}[\arctan(x)]}{\tan ^{2}[\arctan(x)]+1}}} Затем мы используем тождество tan [ arctan ( x ) ] ≡ x {\displaystyle \tan[\arctan(x)]\equiv x}
sin [ arctan ( x ) ] = x x 2 + 1 {\displaystyle \sin[\arctan(x)]={\frac {x}{\sqrt {x^{2}+1}}}} И первоначальная тригонометрическая тождественность Пифагора доказана...
[ arctan ( x ) ] = [ arcsin ( x x 2 + 1 ) ] {\displaystyle [\arctan(x)]=[\arcsin({\frac {x}{\sqrt {x^{2}+1}}})]} y = x x 2 + 1 {\displaystyle y={\frac {x}{\sqrt {x^{2}+1}}}} y 2 = x 2 x 2 + 1 {\displaystyle y^{2}={\frac {x^{2}}{x^{2}+1}}}
x = y 1 − y 2 {\displaystyle x={\frac {y}{\sqrt {1-y^{2}}}}}
x 2 = y 2 1 − y 2 {\displaystyle x^{2}={\frac {y^{2}}{1-y^{2}}}}
y 2 = y 2 ( 1 − y 2 ) y 2 ( 1 − y 2 ) + 1 {\displaystyle y^{2}={\frac {\frac {y^{2}}{(1-y^{2})}}{{\frac {y^{2}}{(1-y^{2})}}+1}}} y 2 = y 2 ( 1 − y 2 ) 1 ( 1 − y 2 ) {\displaystyle y^{2}={\frac {\frac {y^{2}}{(1-y^{2})}}{\frac {1}{(1-y^{2})}}}} Так что это правда: y 2 = y 2 {\displaystyle y^{2}=y^{2}} x = y 1 − y 2 {\displaystyle x={\frac {y}{\sqrt {1-y^{2}}}}}
[ arctan ( x ) ] = [ arcsin ( x x 2 + 1 ) ] = [ arcsin ( y ) ] = [ arctan ( y 1 − y 2 ) ] {\displaystyle [\arctan(x)]=[\arcsin({\frac {x}{\sqrt {x^{2}+1}}})]=[\arcsin(y)]=[\arctan({\frac {y}{\sqrt {1-y^{2}}}})]}
[ arcsin ( x ) ] = [ arctan ( x 1 − x 2 ) ] {\displaystyle [\arcsin(x)]=[\arctan({\frac {x}{\sqrt {1-x^{2}}}})]} [ arccos ( x ) ] {\displaystyle [\arccos(x)]}
cos [ arccos ( x ) ] = x {\displaystyle \cos[\arccos(x)]=x} cos ( π 2 − ( π 2 − [ arccos ( x ) ] ) ) = x {\displaystyle \cos({\frac {\pi }{2}}-({\frac {\pi }{2}}-[\arccos(x)]))=x} sin ( π 2 − [ arccos ( x ) ] ) = x {\displaystyle \sin({\frac {\pi }{2}}-[\arccos(x)])=x} π 2 − [ arccos ( x ) ] = [ arcsin ( x ) ] {\displaystyle {\frac {\pi }{2}}-[\arccos(x)]=[\arcsin(x)]} [ arccos ( x ) ] = π 2 − [ arcsin ( x ) ] {\displaystyle [\arccos(x)]={\frac {\pi }{2}}-[\arcsin(x)]} [ arcsin ( x ) ] {\displaystyle [\arcsin(x)]}
[ arccos ( x ) ] = π 2 − [ arctan ( x 1 − x 2 ) ] {\displaystyle [\arccos(x)]={\frac {\pi }{2}}-[\arctan({\frac {x}{\sqrt {1-x^{2}}}})]} [ arccos ( x ) ] = π 2 − [ arccot ( 1 − x 2 x ) ] {\displaystyle [\arccos(x)]={\frac {\pi }{2}}-[\operatorname {arccot}({\frac {\sqrt {1-x^{2}}}{x}})]} И, наконец, мы имеем [arccos], выраженное через [arctan]...
[ arccos ( x ) ] = [ arctan ( 1 − x 2 x ) ] {\displaystyle [\arccos(x)]=[\arctan({\frac {\sqrt {1-x^{2}}}{x}})]} 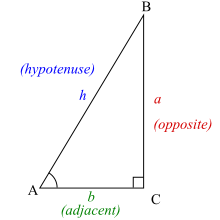












































































































































![{\displaystyle \sin[\arctan(x)]={\frac {x}{\sqrt {1+x^{2}}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7d3ed8cedb4c73b01b65314a15152647c9000086)



![{\displaystyle 1-\sin ^{2}[\arctan(x)]={\frac {1}{\tan ^{2}[\arctan(x)]+1}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d0bb45d076e27715801c0437e364d4b1f45dfb1a)
![{\displaystyle \sin ^{2}[\arctan(x)]={\frac {\tan ^{2}[\arctan(x)]}{\tan ^{2}[\arctan(x)]+ 1}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/31457873262613ebf792de2499d564919e4d18c9)
![{\displaystyle \tan[\arctan(x)]\equiv x}](https://wikimedia.org/api/rest_v1/media/math/render/svg/49ce3dc146ff12eb2efb89a4388afcb984b7da8e)
![{\displaystyle \sin[\arctan(x)]={\frac {x}{\sqrt {x^{2}+1}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5c5222bb21dc2d342d1363c855694bae8b967914)


![{\displaystyle [\arctan(x)]=[\arcsin({\frac {x}{\sqrt {x^{2}+1}})]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/dbc17437ca395ab8ec9e04f2d21096288df5b9a2)







![{\displaystyle [\arctan(x)]=[\arcsin({\frac {x}{\sqrt {x^{2}+1}}})]=[\arcsin(y)]=[\arctan( {\frac {y}{\sqrt {1-y^{2}}}})]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7fbaafc98c5960a737537039cff02fdd72be65da)
![{\displaystyle [\arcsin(x)]=[\arctan({\frac {x}{\sqrt {1-x^{2}}})]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/552a4ab628e13245dc277ab57029427f50ee8ebd)
![{\displaystyle [\arccos(x)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4f61004b1d4ad7ff39f9a7ee50976ec672d2c198)
![{\displaystyle \cos[\arccos(x)]=x}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d9d5691af1ed2de3248e08dbaf90a24a80c1c816)
![{\displaystyle \cos({\frac {\pi }{2}}-({\frac {\pi }{2}}-[\arccos(x)]))=x}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7562bfeec9737b56e7affe3409239315302693c3)
![{\displaystyle \sin({\frac {\pi }{2}}-[\arccos(x)])=x}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5ad20b817a17336b1fc1af8c339a3d7e4cf111f6)
![{\displaystyle {\frac {\pi }{2}}-[\arccos(x)]=[\arcsin(x)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/20f54833eb7dbe561e4cb31e51453ebd14a5cc51)
![{\displaystyle [\arccos(x)]={\frac {\pi }{2}}-[\arcsin(x)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/dfc6a970c74fe335d2b0684f23027e6ca255a9cc)
![{\displaystyle [\arcsin(x)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ef6f2837ad8f82968478d6c5e93863549f833207)
![{\displaystyle [\arccos(x)]={\frac {\pi }{2}}-[\arctan({\frac {x}{\sqrt {1-x^{2}}})]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e6675d621ebaf7c4c4b10adbee9a1fd4b6f1d6eb)
![{\displaystyle [\arccos(x)]={\frac {\pi }{2}}-[\operatorname {arccot}({\frac {\sqrt {1-x^{2}}}{x}} )]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a4fe5f411d564cd54abb12c188ca5885150f1949)
![{\displaystyle [\arccos(x)]=[\arctan({\frac {\sqrt {1-x^{2}}}{x}})]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/13428b7bd426702f18227deb2d8da5f116c16db4)